In Mathematical concepts – context is key I argued that context can provide the challenge, the motivation and the purpose for understanding and using mathematics, and that one approach was to use a problem solving, investigative, open-ended approach—one which uses real materials and situations, and identifies the connections between maths and the real world.
However, teaching mathematics using a problem solving approach, especially one that is based around making connections with the real world, can be demanding—certainly harder than teaching out of a textbook. It requires more planning and preparation in advance if it is going to work successfully.
So, how can you plan what to do and how to link maths with the real world? And what can you do in the classroom and out?
The following flow chart maps out one possible process to use for negotiating and planning what to teach that supports this approach. This process is often best done in collaboration with other teachers – it makes it more creative and you can share the development time.
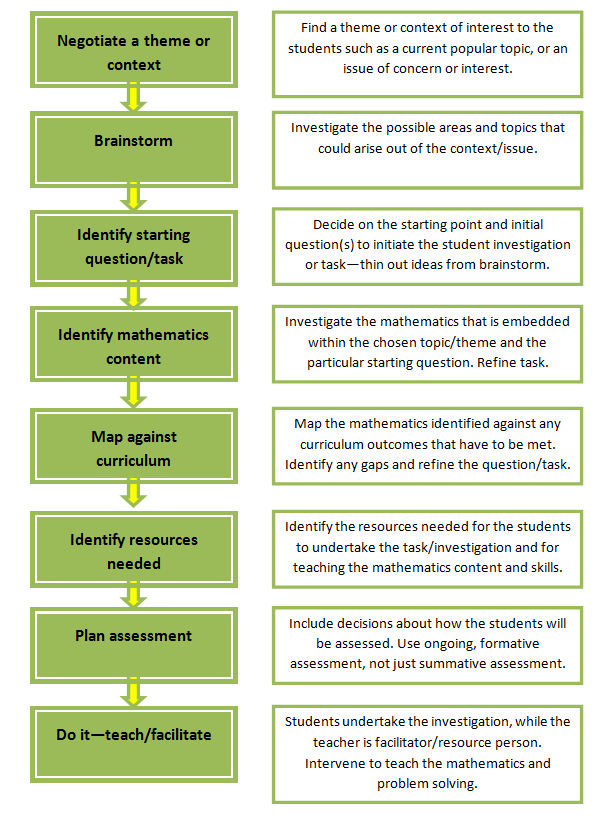
The first and crucial task is to decide what the theme or context is that will be the topic for students to investigate and work on.
Importantly, it should be of interest to the students. It could be a current popular topic, or an issue of concern. It might be about sports, food, travel, cars, holidays, fashion or music. However, it is important to remember that one topic may not be of interest to all students in the group, so a few different options can help.
It can also help if students are involved in the decision making process, so that they can feel ownership in some way. There are several ways to involve students: discussing ideas with them, suggesting topics, brainstorming with them, working in small groups to develop ideas, getting them to write down ideas on cards, and much more.

Image © Shutterstock/Monkey Business Images
Talking with other teachers who teach the same group of students will give you an idea of what the students might be working on in their other classes. If topics are chosen that are related to what students are learning in their other subjects, this provides a cross-curricular approach to teaching and the curriculum.
As teachers, we need to remember that negotiation is a two way process—not just one way from the students' perspective. The teacher is there as an educational leader and has a vital role to play with their knowledge and expertise about what topics are appropriate, what investigations are achievable and manageable, and what mathematics needs to be covered to meet curriculum guidelines.
Stay tuned: In following articles I will illustrate the different stages of the planning process in more detail with examples.
References
Tout, D. and Motteram, G. (2006). Foundation numeracy in context. Melbourne: Australian Council for Educational Research (ACER)
Do you use classroom activities that reflect the real-life situations that your students are likely to face beyond the school gates?
What processes do you use for planning how to use a problem solving approach in your classroom?